Updated: May 10, 2010
We all pass wind occasionally. Even women, although they fervently deny it. Cultural and social issues aside, the combination of our culinary habits, stress and bowel movements result in gas being expelled from our back end into the atmosphere, resulting in some global warming, smell and possibly some rocket-like lift.
How much exactly, the astute and curious among you may ask. Well, this is what we're going to find out today. We will examine the delicate aspects of physics involved, including lift, pressure, velocity, and maybe even branch into complicated stuff like Bernoulli, stagnation effects and nozzle shape. I may even hazard to guess the speed and lift of a typical fart by its acoustic signature. Follow me.
Bowel physics
To be able to calculate the lift force, we will require some basic data. First and foremost, the pressure generated in our colon. We cannot assume it's anything major. Still, it's higher than typical air pressure, which is around 1 bar on average. I'd say 1.5 bars, although this is probably too much. Pressure will determine the velocity and ultimately the duration of an event, although muscle contraction can keep a lid on the pot. We all do this during long business meetings.

Next, we need the mass of the expelled gas. This too, will determine the duration of the fart event. Combined with the pressure, which is determined by how bloated we feel, e.g. how painful the pre-event sensation is, as well as the shape of the keester, the fart mass will be used to calculate the lift force.
To wit, air contained in the colon does not take much space. Probably the last 40-50 cm of it. Assuming an average diameter of about 4 cm, then in total, we have about 630 cubic cm of air, pressurized to 1.5 bar, or roughly 950 cubic cm of air. Air density at room temperate is about 1.2kg/m3, which means only 0.0012 gr per cubic cm. In total, the gas in our bowel has a mass of only about 1.15 gr.
Now what about the speed?
We did talk about this a little in our Honey article, but basically, Flow = Area x Velocity. In other words, we can derive velocity from the flow and the keester area. Let's assume the typical fart lasts 1 second. The flow we have, in cubic meters is: 0.00095 m3/sec. The last missing bit is the area of the orifice. This is pure pseudo-science, but I'll go with something like a dime cross-section, sideways, which means something like 1 x 0.1 cm, or 0.00001 m2.
Dividing the two we get:

So we have a rather lovely velocity of 95 m/s! That's quite a lot actually!
Let there be lift!
To calculate lift, we will use the classic equation:

Where ρ is air density, υ is the true airspeed, A is the planform area and C is the lift coefficient, which is a very complex figure derived from Mach number, Reynolds number and the Angle of Attack (AoA). We're really getting super physical here.
We will simplify things a little. Lift coefficient can go from anywhere from 0.2 to 2, more or less. Let's assume 1. But there's not way of knowing the platform area. We don't have any wings. And we don't have any forward velocity, so to speak. We're more like a rocket. So thrust it is!
In thrust we trust
Thrust is very simple to calculate. It's Flow x Velocity. T = F x V = 0.9N. So our weight (mass) is reduced by about 90 gr. Of course, any angle deviation from vertical reduces the effective thrust. This means that we're lightest when seated and having a petard moment.

In which case, our equation becomes:
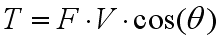
My calculations could be wrong, but does it really matter?
Note: As you can see, the velocity will change significantly with the duration of the event, as well as the quantity of gas. But if we assume the bowel always contains pretty much the same quantity, this explains why the rather silent, quick farts smell more - because of the higher velocity, they spread to greater distances.
What would it take to fart levitate?
A stellar question! Let's assume that our body can withstand the pressure. What we need is approx. 750N of thrust to negate the effect of gravity on an average-size average-weight person and get them airborne. We need 750 times more than we currently have.
To achieve this, we either require flatulence that is 750 times faster than it already is, which seems rather impossible. First, at close to 100 m/s, we're already fast. At just 3.5 times more than that, we would break the sound barrier and go supersonic. At 750 times more, we'd be traveling at Mach 250, which seems impossible inside the atmosphere. Frankly, it exceeds any man-made projectile.
So we need to focus on the Flow. We need 750 times more than we currently have. Our bowels, currently pressurized at 1.5 bar, would have to sustain the pressure of 1125 bars, which is equal to that 11.25 km below the sea. This also means we would be holding 750 times more gas in our colon, to a total mass of about 850 gr.
More numbers
We have a projectile with a mass of 850 gr expelled at 95 m/s from our body. The kinetic energy of this missile is 7.6KJ. If the fart lasts one second, then it generates 7.6KW of power. This would be ten times more than Bugatti Veyron, albeit for a very short period.
Now, focusing on Newton's Third Law of Motion, which is basically the conservation of momentum, were our big fart to slam into a still 75-kg body and transfer all of its energy/momentum into it, then we would have the big body gaining velocity of about 1.08 m/s. We would be flying upwards very respectably!

Finally, the sounds of silence
Are you well familiar with the smell is inversely proportional to the sound claim? Well, it could be true. It's all about velocity and frequencies. Is there any relation between the two? We will assume that the diameter of the aperture (keester) is what dictates the wavelength. In theory, the smaller it is, the higher the frequency, the more pitched the sound. Therefore, we have:

Where υ is the air velocity and λ is the diameter of the aperture. On the first glance, it seems there's no relation between » and the fart speed. But there is. Because, as we've seen earlier, the so-called valve area dictates the velocity of the released gas. Given a constant mass of cabbage-flavored particles, we can derive the audio frequency of the event. Taking numbers we used before, the typical 1-second event will resonate at:

We get 330KHz, this is way above the human hearing levels. So we must assume that there's a significant stagnation effect, plus the aperture cross-section changes dynamically. Henceforth, we must assume that most farts are indeed slower than we expect. But the general theory aligns well with the popular saying.
The silent ones (with very high frequencies) are indeed the smelliest. Me rules!
Conclusion
I think you've just come out enlightened. While this topic may not appear important, it definitely has its merit. First, discussing the physics thereof can be a supreme ice breaker or an opening line when you hit on someone in a bar. Furthermore, it gives you a certain intellectual edge, because people may really believe you.
Well, that would be all for today. We now know that human-powered aircraft are not meant to be. We also know the little bits about what's happening after lunch. Most importantly, we have proved the popular saying that ties flatulence sound effects to olfactory disturbances.
Take care, brave people!
Cheers.